Angles are an essential concept in mathematics and geometry. They help us understand the relationship between lines and shapes, and how they interact with each other. One particular type of angles that is often encountered is alternate angles. In this blog post, we will explore what alternate angles are, why they are important, and how they can be used in various geometric situations.

Definition

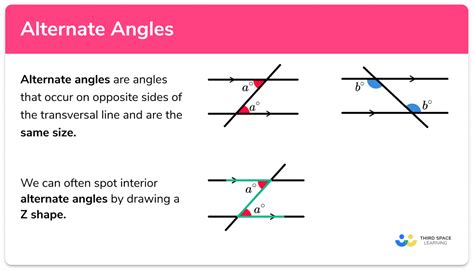
Alternate angles, also known as “alternate interior angles,” are a pair of angles formed when a transversal (a line that intersects two or more other lines) crosses two parallel lines. These angles are located on opposite sides of the transversal and inside the pair of parallel lines. In simpler terms, alternate angles are a pair of angles that are not adjacent but lie on different lines on the same side of a transversal.

Properties

One of the key properties of alternate angles is that they are congruent, meaning they have the same measure or size. This property makes alternate angles useful in solving various geometric problems and proving theorems. Additionally, alternate angles are always formed when parallel lines are intersected by a transversal, regardless of the angles involved.

Example

Let’s consider a practical example to better understand alternate angles. Imagine two parallel lines, line A and line B, intersected by a transversal line C. If angle 1 and angle 2 are alternate angles, then angle 1 is congruent to angle 2. The same applies to angle 3 and angle 4, and so on. This congruence property allows us to make calculations and deductions about the angles involved in a given situation.

Applications

Alternate angles find applications in various real-world scenarios. For instance, in architecture and construction, understanding alternate angles helps in designing structures with parallel lines, such as windows and doors. Engineers use alternate angles to ensure precise measurements and alignments. Additionally, alternate angles play a crucial role in navigation systems, as they assist in determining direction and orientation.

Mathematical Theorems

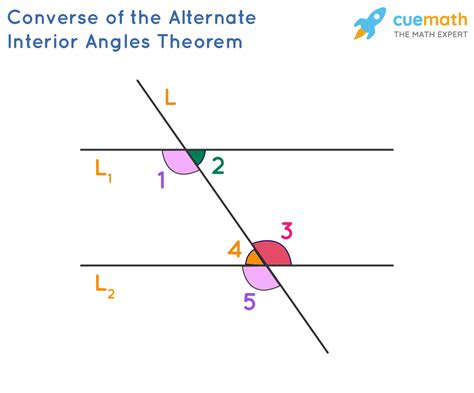
Alternate angles are an essential component in proving several theorems in geometry. One such theorem is the “Alternate Interior Angles Theorem,” which states that if a transversal intersects two parallel lines, then the alternate interior angles are congruent. This theorem has significant implications for solving geometric problems and forming logical arguments.

Conclusion

Alternate angles provide a valuable tool for understanding and solving geometric problems involving parallel lines intersected by a transversal. Their congruence property and various applications make them an essential concept in mathematics, engineering, and architecture. By recognizing and utilizing alternate angles, we can unlock new insights and solve complex problems in the world of geometry.

Leave a Comment

We hope this blog post has provided you with a clear understanding of alternate angles and their significance. If you have any questions, examples to share, or additional insights, please leave a comment below. We would love to hear from you!